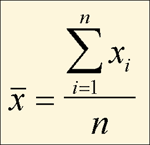
Means are measures of a central tendency. In this section, you will learn about three types of means:
The finite population mean of X1 , X2 ,…. XN is defined as the sum of the values Xi divided by the population size N. Typically, in a non-survey setting an arithmetic mean is estimated by taking a simple random sample of the finite population, x1, x2,…,xn, summing the values and dividing by the sample size n.
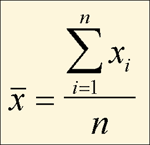
This is often referred to as the arithmetic mean. On average, the result of the arithmetic mean would be expected to equal the result of the population mean.
For NHANES 1999-2002 a sample weight, wi, is associated with each sample person. The sample weight is a measure of the number of people in the population represented by that person. For more information on sample weights, please see the Weighting module. To obtain an unbiased estimate of the population mean, based on data from the NHANES 1999-2002 sample, it is necessary to take a weighted arithmetic mean.

In instances where the data are highly skewed, geometric means can be used. A geometric mean, unlike an arithmetic mean, minimizes the effect of very high or low values, which could bias the mean if a straight average (arithmetic mean) were calculated. The geometric mean is a log-transformation of the data and is expressed as the N-th root of the product of N numbers.