Case Study: Plotting and interpreting BMI-for-age using the Metric system
A Case Study of ‘Charlene’
Step 1. Obtain accurate weights and stature measurements
Charlene, a girl, comes in for a visit.
Here is Charlene's basic information:
Date of Birth (DOB): 10/30/96
Date of Visit (DOV): 11/5/00
Weight = 18.3 kg
Height = 103.5 cm
Step 2. Select the appropriate growth chart
Based upon the above information, select a growth chart:
That's correct!
Because Charlene is a normal 4-year old, a standing height was obtained. The appropriate chart to use is the ‘Girls 2 to 20 BMI-for-age' chart.
Step 3. Record the data
The data entry table on the clinical growth chart can be completed with information relevant to the growth chart. Enter the missing data on the Data Entry Table and then click the 'Submit' button.
Charlene
Date of visit: 11/05/00
Child's age: 4
Weight: 18.3 kg
Height: 103.5 cm
Step 4. Calculate BMI
Now calculate BMI at the time of Charlene's clinic visit.
Date of visit: 11/05/00
Child's age: 4
Weight: 18.3 kg
Height: 103.5 cm
BMI = (weight / height / height) x 10,000
BMI = (18.3 kg/103.5 cm/103.5 cm) x 10,000 = 17.1
Enter the BMI on the Data Entry Table and click 'Submit':
| Date of visit | Age | Weight | Height | BMI |
|---|---|---|---|---|
| 11/05/00 | 4 | |||
That's right.
Step 5. Plot measurements
| Charlene | ||||
|---|---|---|---|---|
| Date of visit | Age | Weight | Height | BMI |
| 11/05/00 | 4 | 18.3 | 103.5 | 17.1 |
All of the necessary information is recorded and Charlene's BMI can be plotted. On the BMI-for-age chart, find Charlene's age on the horizontal axis and visually draw a vertical line up from that point. Then find her BMI on the vertical axis and visually draw a horizontal line across from that point. The point where the two intersect represents Charlene's BMI-for-age.
Click on the correctly plotted BMI-for-age chart from the three options below:
That's the correct chart!
When plotted on the growth chart, Charlene's BMI-for-age falls between the 85th and 90th percentile curves.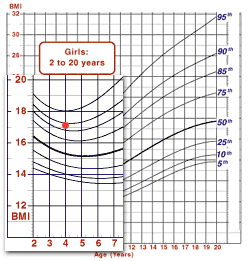
Step 6. Interpret the plotted measurements
Since Charlene's BMI-for-age falls between the 85th and 90th percentile curves, this means that of 100 children with the same gender and age as Charlene, 85 to 90 children will have a BMI-for-age lower than Charlene's and 10 to 15 children will have a BMI-for-age greater than hers. Because Charlene's BMI-for-age is above the 85th percentile, and lower than the 95th percentile, she is...
That's correct!
Since Charlene's BMI-for-age falls between the 85th and 90th percentile curves, she is overweight.
Congratulations! You have successfully calculated, plotted and interpreted Charlene's BMI-for-age.
Thank you for completing the module. Click here to visit the references and resources page, or click below to return to the Main Modules page.
- Page last reviewed: May 9, 2014
- Page last updated: May 9, 2014
- Content source:


 ShareCompartir
ShareCompartir